Milan Kornjača, Rhine Samajdar, Tommaso Macrì, Nathan Gemelke, Sheng-Tao Wang, Fangli Liu

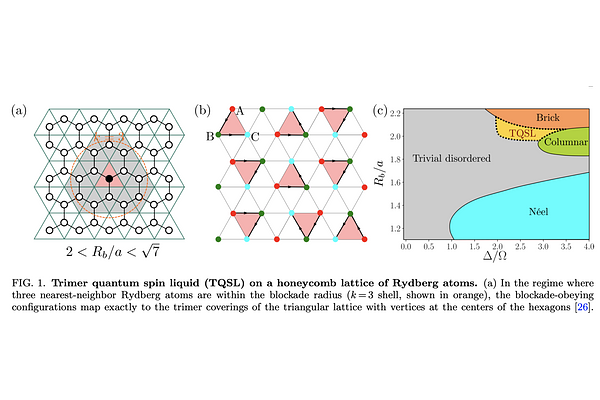
Quantum spin liquids are elusive but paradigmatic examples of strongly correlated quantum states that are characterized by long-range quantum entanglement. Recently, the direct signatures of a gapped topological $\mathbb{Z}_2$ spin liquid have been observed in a system of Rydberg atoms arrayed on the ruby lattice. Here, we illustrate the concrete realization of a fundamentally different class of spin liquids in a honeycomb array of Rydberg atoms. Exploring the quantum phase diagram of this system using both density-matrix renormalization group and exact diagonalization simulations, several density-wave-ordered phases are characterized and their origins explained. More interestingly, in the regime where third-nearest-neighbor atoms lie within the Rydberg blockade radius, we find a novel ground state -- with an emergent $\mathrm{U}(1)\times \mathrm{U}(1)$ local symmetry -- formed from superpositions of classical {\it trimer} configurations on the dual triangular lattice. The fidelity of this trimer spin liquid state can be enhanced via dynamical preparation, which we explain by a Rydberg-blockade-based projection mechanism associated with the smooth turnoff of the laser drive. Finally, we discuss the robustness of the trimer spin liquid phase under realistic experimental parameters and demonstrate that our proposal can be readily implemented in current Rydberg atom quantum simulators.